Convex polyhedra
Archimedean solids are convex polyhedra that have a similar arrangement of nonintersecting regular convex polygons of two or more different types arranged in the same way about each vertex with all sides the same length (Cromwell 1997).
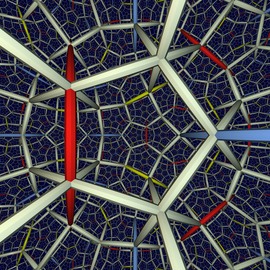
In this visualization, convex polyhedra and truncated icosidodecahedra were constructed according to the Hart & Kaplan method by placing regular polygons at the rotational axes of a polyhedral symmetry group.
The various geometric shapes were created and manipulated in a vector graphic program and set on a background composed of thousand colored blocks representing a computer file system, each rectangle size being proportional to the file’s size.
The blocks were sliced, rearranged and given a perspective or Droste effect to add to the dynamic of the final composition.

Convex polyhedra and truncated icosidodecahedra constructed following the Hart & Kaplan method by placing regular polygons at the rotational axes of a polyhedral symmetry group.
The various geometric shapes were created and manipulated in a vector graphic program and set on a background composed of thousand colored blocks representing a computer file system, each rectangle size being proportional to the file’s size.
The blocks were sliced, rearranged and given a perspective or Droste effect to add to the dynamic of the final composition.