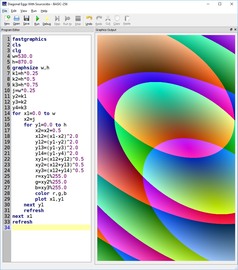
jReality Screenshots
galeri
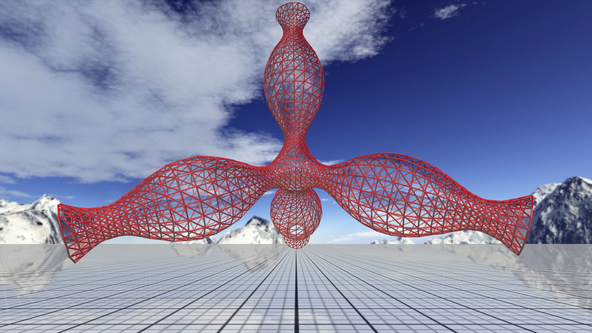
jReality Screenshots
Screenshots from the program jReality Exhibit.

Matheon Bear
The sculpture of the Matheon Bear stands in front of the Maths building of the TU Berlin. The bear is interesting from a mathematical point of view, because of the pattern painted on it. The periodic pattern of circles and Matheon logos filling the plane forms the basis. The mathematical challenge was to apply this pattern such that the forms distorted as little as possible. Actually, you can see that all of the angles appearing in the pattern also appear on the bear. We can say that the image of the pattern on the bear is “conformal”.
Lisans CC BY-NC-SA-3.0