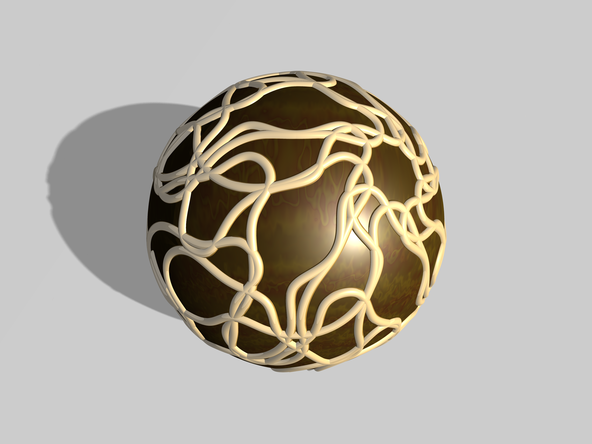
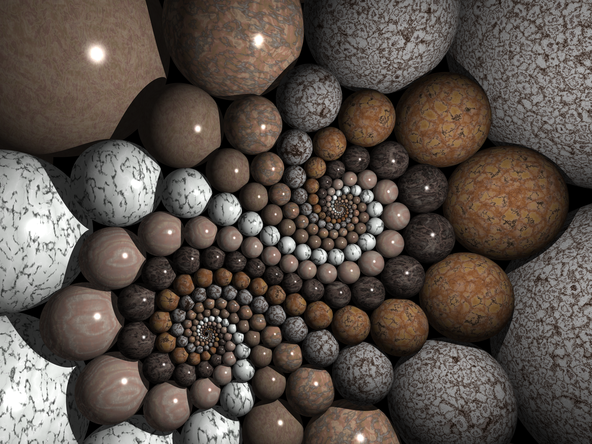
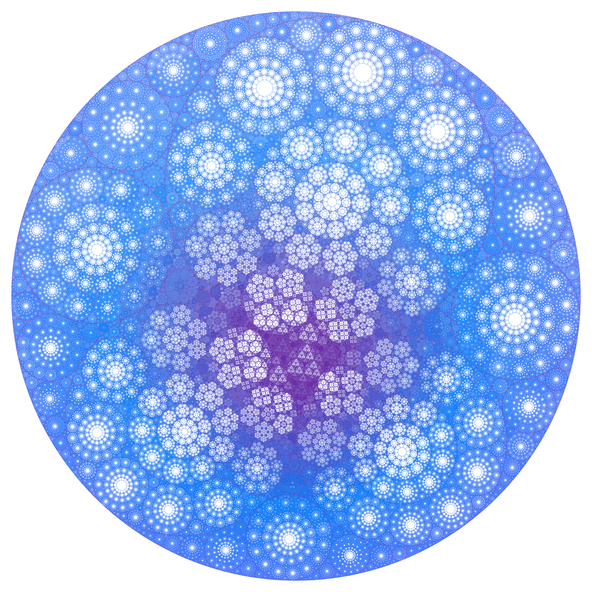
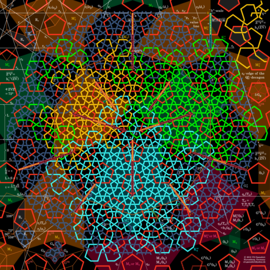
My contributed pictures for the Imaginary / NIMS exhibition at ICM Seoul 2014
Resimlerimden bazıları Imaginary ekibi tarafından Seul ICM sergisinde gösterilmek üzere seçildi.
Burada açıklamalarıyla birlikte bunları görebilirsiniz. Sergide diğer kullanıcılardan seçilmiş resimler de gösterildi.

Apophysis
In 1988, Michael Barnsley developped the theory of Iterated Function Systems (IFS in short), a way of describing and generating fractals well suited for programming. The functions used were all affine transforms, and can generate objects like ferns, Sierpinski gaskets… Apophysis (www. aphophysis.org) is a computer program, initiated by Scott Draves, which generalize IFS by using non linear functions instead of affine ones. Apophysis interface is quite complete and user friendly, allowing easy and fast experiments. This program is a good illustration of what experimental mathematics can offer : fast tests, large universe of possibilities.