The Heidelberg Laureate Forum Foundation invites you to visit the exhibition “Mathematics of Planet Earth” at Karlstorbahnhof in Heidelberg, Germany! It runs from July 5 to Aug 2, 2015, for the first time in Heidelberg. It offers a big variety of exciting adventures for children and adults alike.
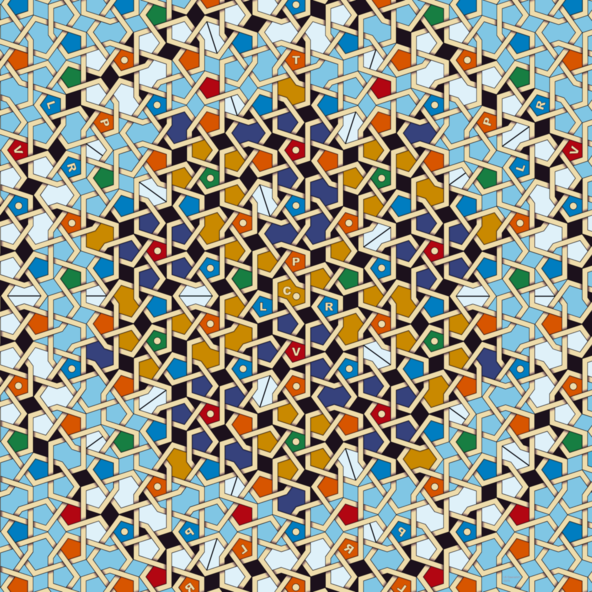
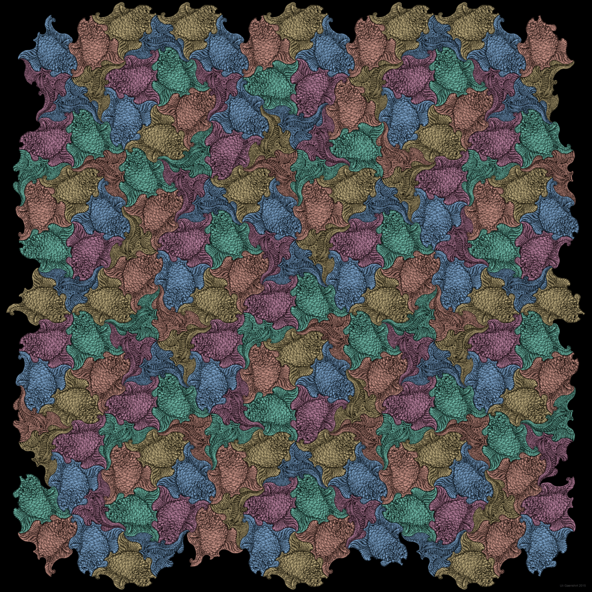
Quasicrystalline Wickerwork
In 1982, Dan Shechtman discovered a nuclear structure with fivefold rotational symmetry. Today, nuclear structures of this type are called quasicrystals.
In general, growth models for decagonal quasicrystals with tenfold or fivefold rotational symmetry are based on geometrical, quasiperiodical tilings which were found by Roger Penrose in 1973.

Quasicrystalline Wickerwork
Although the wickerwork in the foreground of the large graphic is composed of medieval girih tiles (Persian girih, Eng. knot), it has a relationship to the atomic structure of a decagonal quasicrystal.
Adapting the girih tiles to a modern rhombic Penrose tiling they are generating a girih wickerwork with a good approximation to a tenfold rotational symmetry. The resulting closed nodes (green, yellow and blue) surprisingly correspond to the geometry of a cover model commonly used today, which can be seen in the background of the wickerwork.
For a detailed description of the picture please refer to the pdf-documents (GERMAN/ENGLISH) provided below and to the files below the image.
This picture, like some others from this gallery, is part of the exhibition “IMAGINARY - Form und Formel mathematischer Fantasie” which was first shown in June 2016 in Nuremberg.
(www. imaginary.org/event/imaginary-in-nuremberg)
(www. imaginary.org/event/imaginary-at-sigena-gymnasium-in-nuremberg)
(www. imaginary.org/gallery/hand-made-hands-on)
(https://patterninislamicart.com/s/collections/main-archive/piia_image/tu…) Girih pattern in Kayseri