IMAGINARY in Kaohsiung, Taiwan
evento
9 AM - 5 PM
Créditos
- Idea
Sitio web
IMAGINARY is coming to Taiwan! The first exhibition will take place at the National Science and Technology Museum (NSTM) in Kaohsiung, Taiwan. The exhibition demonstrates a variety of mathematical content from IMAGINARY, including interactive programs, picture galleries, films and 3D sculptures. Moreover, we invite some experts in Taiwan to contribute their works combining mathematics and arts to the exhibition. The exhibition will travel through Taiwan afterwards. The next stop is supposed to be Taipei.
(video credits: Narrator: 劉懿婕 (Demi Liu), Director: 陳宏賓 (Hongbin Chen), Producer: 鍾冠毅 (Kuan-I Chung), Co-organizer: Department of Applied Mathematics, National Sun Yat-sen University, Organizer: TMS)














王偉仲、高欣欣 (from the left)

陳訓祥、陳榮凱、郭嘉真 (from the left)
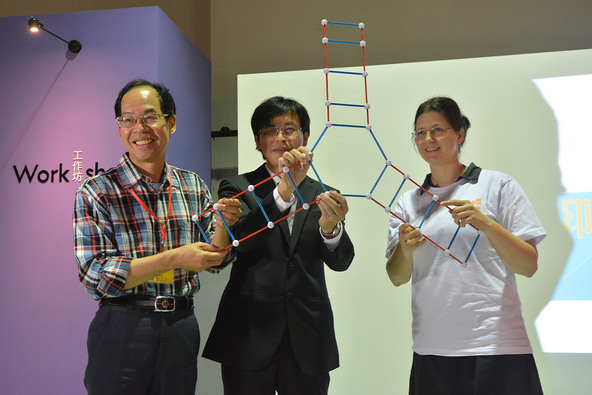
陳訓祥、陳榮凱、Bianca Violet (from the left)
Logo of IMAGINARY Taiwan
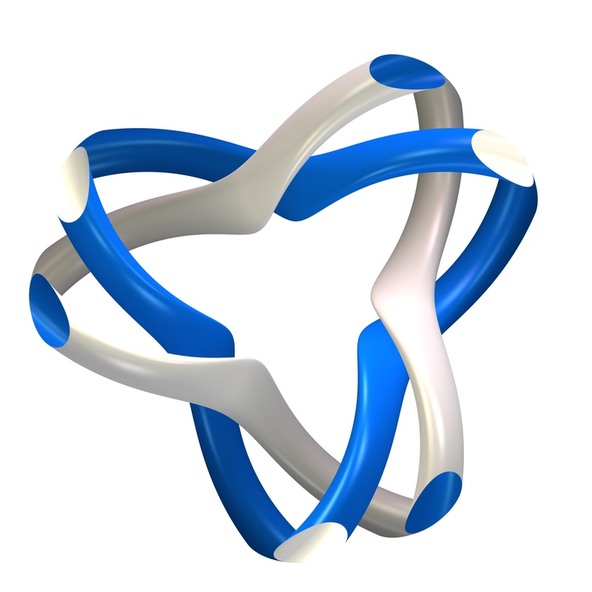
This is a detail of a pair of solid trefoil knots.
A trefoil knot is basically a closed curve - there are no ends to tie or untie - and it can be obtained by joining the two loose ends of a common overhand knot. You cannot untie it in three dimensions without cutting, which makes it a nontrivial knot. It is actually the simplest nontrivial knot.
The trefoil knot is named after the three-leaf clover (or trefoil). There are two variants: the left-handed and the right-handed trefoil knot, and it is not possible to continuously deform one into the other. This property is called chirality.
In knot theory, the trefoil knot can be classified as a (2,3)-torus knot. It lies on the surface of an unknotted torus in three-dimensional Euclidean space, winding two times around the interior circle line of the torus and three times around its axis of rotational symmetry.
A solid trefoil knot is the boundary surface of a small smooth tubular neighborhood of the trefoil knot curve.
Find out more about how to create solid trefoil knots with the SURFER software here.



Archivos
Todos los eventos
- 1 de 32
- siguiente ›
