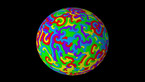
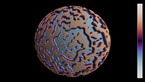
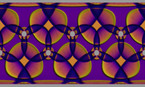
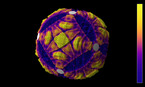
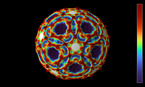
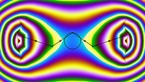
Waves with dodecahedral symmetry on the sphere, 2d projection
película
Licencia
Créditos
Solution of the wave equation on a sphere. Reflecting obstacles have been placed around the vertices of a regular dodecahedron. The initial state is a set of circular waves concentrated at the centers of the faces of the dodecahedron. The video shows an equirectangular projection.
The simulation shows a solution of the wave equation on a sphere, obtained by a finite difference scheme. There are Dirichlet boundary conditions on a set of discs of constant radius placed on the vertices of a regular dodecahedron. The initial state is a set of circular waves concentrated near the centers of the faces of the dodecahedron, which form a regular icosahedron.
The point of view rotates around the sphere in the course of the simulation. Part 1 shows the wave height, while part 2 shows the energy averaged from the beginning of the simulation.